koobas.hobune.stream
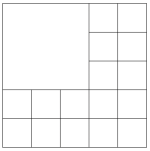
A Pattern Puzzle for the New Year
Over at the Visual Patterns site, the directions state that if you click on a pattern, you’ll get to see the number of objects in the 43rd step. Why 43? I assumed that it had to do with Fawn Nguyen being a fan of Troy Polamalu — which, as far as I’m concerned, would be just one more reason to have an infinite amount of respect for her — but when I asked about it, Fawn explained that 43 was chosen as…
…a random number that was farther down the step number to prevent students from finding the number of objects recursively, but not too far.
This explanation sits well with my beliefs. In my book One-Hundred Problems Involving the Number 100, I stated that it’s appropriate to ask students to find the 100th term in a sequence because 100 is “big enough to exhilarate, but not so big as to intimidate.” The same could be said about 43.
Following Fawn’s lead, here’s a problem to get you in the spirit for the new year. Feel free to share this problem with your students on or near January 1.
How many squares would be in the 43rd element of this sequence?
Coincidentally, I shared this sequence with Fawn, and it now appears as #392 on the Visual Patterns site.
Speaking of sequences, here’s my favorite infinite sequence joke.
Infinitely many mathematicians walk into a bar. The first says, “I’ll have a beer.” The second says, “I’ll have half a beer.” The third says, “I’ll have a quarter of a beer.” They continue like this, each one ordering half as much as the last. The barman stops them and pours two beers. One of the mathematicians says, “That’s it? That’s not enough for all of us!” The bartender replies, “C’mon, folks. Know your limits.”
For fun, figure out how much beer the 43rd mathematician asked for.
And as a little more fun, guess the value of all the coins in the glass below. As a hint, there are the same number of quarters, dimes, and nickels, but three times as many pennies as dimes. (Said another way, Q:D:N:P::1:1:1:3.)
If you think about it a little, you’ll realize the answer without doing any computation.
Happy New Year!
Mathy Zoom Backgrounds
Do you seek the admiration of your colleagues or the respect of your students?
Do you wish to create the illusion that you’re funny and cool?
Do you long to be the envy of your virtual social circle?
Unfortunately, you’re reading a math jokes blog, which means there may not be much hope for you. But a possible start may be to download some of the math joke backgrounds below for your next online meeting. I’ve been using them for the past few weeks, and I don’t think it’d be an overstatement to say that I’m now the envy of the internet. I mean, I’ve got a face for radio, but you have to admit that I look pretty fantastic when there’s a math poem above my head and equations on either side of it:
And guess what? You can look that cool, too!
To use any of the images below, simply right click and “Save Image As…,” then install them as virtual backgrounds (Zoom, Google Meet). If you’d like a better look at any of them before deciding if they’re worth valuable memory on your laptop, just click on an image to open it full screen.
Opinion Minus Pi
Trig Tank
Binary
Root Beer
Punch Line
Pi and E
Pentagon, Hexagon, Oregon
Tom Swiftie
Graph Paper
Complex Person
6 Afraid of 7
Math and Coffee
KenKen 12 Puzzle for 12/12
Today is the twelfth day of the twelfth month, and in honor of the date, here’s a 4 × 4 KenKen puzzle that has 12 as the target number in each cage. The entire puzzle has only four cages, and it only uses addition and multiplication. Have at it!
But a post with just a KenKen puzzle isn’t much of a post, especially on a math jokes blog. So let’s consider some jokes that have to do with the association between 12 and a dozen. The following are some mathematical insults you can use if you’re playing the dozens.
Yo momma is so fat, she’s proof that the universe is expanding exponentially.
The shortest distance between two points is around yo momma’s ass.
Yo momma is so fat, her volume is an improper integral.
Yo momma is so crazy, when she received a can of Pepsi from the vending machine, she started jumping up and down, yelling, “I won! I won!”
Yo momma is so dumb, she thinks convex are inmates locked in a prism.
Yo momma is so infinitely fat, she can eat as much as she wants and not gain any weight.
Yo momma thinks cosine is what she does for a loan.
Yo momma is so dumb, she sleeps with a ruler to keep track of how long she sleeps.
Yo momma is so fat, she took geometry because she heard there was gonna be π.
Yo momma is so fat, the ratio of her circumference to diameter is 4.
Yo momma is so fat, in a love triangle she’d be the hypotenuse.
Yo momma thinks coincide is what you should do when it’s raining.
The integral of your mom is fat plus a constant, where the constant is equal to more fat.
Yo momma is so dumb, she doesn’t know the difference between a doughnut and a coffee cup.
Yo momma is so dumb, she thinks crossing a mosquito and a mountain climber yields |mosquito| × |mountain climber| × sin(θ).
The derivative of yo momma is strictly positive.
Yo momma is so dumb, she serves beer in Klein bottles.
Yo momma is so dumb, she thinks that if two people go into a hotel and three come out, the first two must have pro-created.
Yo momma is so dumb, she can’t even solve a second‑order non‑homogeneous differential equation.
Yo momma is so fat, her dress size requires an exponent.
The limit of yo momma’s ass tends to infinity.
Yo momma is so fat, when she steps on the scale, it displays π without a decimal point.
Yo momma’s muscle-to-fat ratio can only be explained by irrational complex numbers.
Yo momma is so ugly, Pythagoras wouldn’t touch her with a 3-4-5 triangle.
Guess the Graph
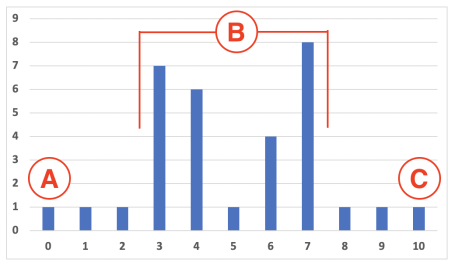
The bar graph below was created because of a recent discussion with my wife. The title and axis labels have been removed. Can you identify the data set used to create the graph? I’ll give you some hints:
- The data set contains 32 elements.
- It’s based on a real-world phenomenon from this year.
- The middle five categories account for 81% of the data.
- The special points marked by A, B, and C won’t help you identify the data set, but they will be discussed below.
Got a guess?
No clue? Okay, one more hint:
- The vertical axis represents “Teams.”
Still not sure? Final hints:
- Point A represents the lowly J-E-T-S, who are currently winless.
- The region outlined by B shows that 26 teams have from 3 to 7 wins.
- Point C on the graph represents my Pittsburgh Steelers, whose record is a perfect 10‑0. (It’s my hope that I’ll still be able to gloat on Friday morning, after the Steelers host the Ravens on Thanksgiving night.)
This graph was generated while discussing the current standings in the NFL with my wife, who speculated that there seemed to be a lot of really good teams and a lot of really bad teams this year. The horizontal axis represents the number of wins. As it turns out, the distribution above is somewhat typical at this point in the season. At the end of most seasons, about 2/3 of the teams finish a 16-game season with 5 to 10 wins. It may be a little unusual that there are 8 teams with 7 wins, but it’s not statistically cray-cray.
If you’ve read this far, then you may enjoy these other math-related football trivia questions:
- Describe two ways in which an NFL game can end with a score of 2‑0.
- What’s the greatest score that cannot be attained by scoring only touchdowns (7 points) and field goals (3 points)?
- Express the ratio of width:length of a football field. For length, include the end zones.
- What are the only positions allowed to wear single-digit uniform numbers?
- During a typical broadcast of an NFL game, approximately what percent of the time is spent actually playing football (as opposed to commercials, half time, or just milling around between snaps)?
Happy Drinksgiving! And, go Stillers!
—
Answers
- A game can end 2‑0 if one team scores a safety and the other team doesn’t score at all. It can also end 2‑0 if one team forfeits before either team has scored, by league rule. (In high school and college, a forfeit is officially recorded as a 1‑0 loss.)
- 11 points. Any point total above that is (theoretically) possible. Below that, it’s not possible to score 1, 2, 4, 5, or 8 points.
- A field is 53 1/3 yards wide and 120 yards long. In feet, that’s 160:360, which can be reduced to 4:9.
- Quarterbacks and kickers.
- According to several analyses, 11 minutes of a three-hour broadcast is spent actually playing. That’s about 3%. Sheesh.
Mathy Portmanteaux
The term portmanteau was first used by Humpty Dumpty in Lewis Carroll’s Through the Looking Glass:
Well, ‘slithy’ means “lithe and slimy” and ‘mimsy’ is “flimsy and miserable.” You see, it’s like a portmanteau — there are two meanings packed up into one word.
Interestingly, the word portmanteau itself is also a blend of two different words: porter (to carry) and manteau (a cloak).
Portmanteaux are extremely popular in modern-day English, and new word combinations are regularly popping up. Sometimes, perhaps, there are too many being coined. In fact, one author refers to these newcomers as portmonsters, a portmanteau of, well, portmanteau and monster that attempts to capture how grotesque some of these beasts are. An abridged list of portmonsters would include sharknado, arachnoquake, blizzaster, snowpocalypse, Brangelina, Bennifer, Kimye, Javanka, fantabulous, and ridonkulous.
These are Portman toes, not portmanteaux.
Portmanteaux seem to proliferate most easily in B-movie titles, weather, and celebrity couples, but the world of math and science is not free from them. Here are a few mathy portmanteaux, presented, of course, as equations.
ginormous = giant + enormous, really big
guesstimate = guess + estimate, a reasonable speculation
three-peat = three + repeat, to win a championship thrice
clopen set = closed + open set, a topological space that is both open and closed
bit = binary + digit, the smallest unit of measurement used to quantify computer data
pixel = picture + element, a small area on a display screen; many can combine to form an image
voxel = volume + pixel, the 3D analog to pixel
fortnight = fourteen + night, a period of two weeks
parsec = parallax + second, an astronomy unit equal to about 3.26 light years
alphanumeric = alphabetical + numeric, containing both letters and numerals
sporabola = spore + parabola, the trajectory of a basidiospore after it is discharged from a sterigma
gerrymandering = Elbridge Gerry + salamander, to draw districts in such a way as to gain political advantage (In the 1800’s, Governor Elbridge Gerry redrew districts in Massachusetts to his political benefit. One of the redrawn districts looked like a salamander.)
megamanteau = mega + portmanteau, a portmanteau containing more than two words, such as DelMarVa, a peninsula that separates the Chesapeake Bay from the Atlantic Ocean and includes parts of Delaware, Maryland, and Virginia
meganegabar = mega + negative + bar, the line used on a check so that someone can’t add “and one million” to increase the amount
(By the way, when Rutgers University invited Jersey Shore cast member Snooki Polizzi to speak to students on campus in 2011, they paid her $32,000, which is $2,000 more than they paid Nobel and Pulitzer Prize winning author Toni Morrison to deliver a commencement address six weeks later.)
Getting Back to My Roots
For years, this blog represented the finest mathematical humor that the internet had to offer. That hasn’t been the case so much recently, so it’s time I got back to my roots — of course, for me, those would be cube roots…
I was inspired to craft this post of horrendously bad puns when my sister’s friend shared this photo with me:
And I figured if I have to suffer, you should, too.
How many math grad students does it take to change a light bulb? Just one, but it takes nine years.
What’s the best tool for math class? Multi-pliers!
Think outside the regular quadrilateral.
When asked how good she was at algebra, the student replied, “Very able.”
What’s the difference between the radius and the diameter? The radius.
Are you depressed when you think about how dumb the average person is? Well, I’ve got bad news for you… nearly half the population is even dumber.
How do you make one disappear? Add a g, then it’s gone.
Writing haiku is
tough, because you have to count.
Writers don’t like math.
Light travels faster than sound. This is why some people appear bright until you hear them speak.
The grad student had trouble getting the pizza box into the recycling can. It was like trying to put a square peg in a round hole.
How is the moon like a dollar? Both have four quarters.
Don’t look now, but there’s a suspicious man over there with graph paper. I think he’s plotting something.
Thinking > Symbols
As if having to wear a mask while flying isn’t scary enough, last week I had a harrowing experience.
See Valentin Kirilov’s full video at https://vimeo.com/156775315.
It’s normally a three-hour flight from Yellowknife in the Northwest Territories to Portland, but about 20 minutes into a recent flight, there was an unsettling noise followed by an announcement from the pilot: “Ladies and gentlemen, this is your captain. Please don’t be alarmed by the noise you just heard near the left wing. Let me assure you that everything is okay. We’ve lost an engine, but the other three engines are working just fine. They’ll get us to Portland safely. But instead of taking 3 hours, it’s going to take 4 hours.”
She said this matter-of-factly, as if she were commenting on the color of my shirt or pointing to a squirrel that was enjoying an acorn. It was reassuring that she didn’t seem worried — but still, we had lost an engine! The cabin grew quiet as we waited to see what would happen next. But, nothing. We continued to fly as if nothing had happened, and the din of conversation slowly resumed.
And then there was another noise near the right wing, followed by the pilot: “Ladies and gentlemen, this is your captain again. Unfortunately, we’ve lost another engine. But the two remaining engines are functioning properly. We’re going to get you to Portland safely, but now it’s gonna take about 6 hours.”
Needless to say, you could hear a pin drop as we passengers waited with baited breath. And sure enough, there was another noise, and the pilot again: “Ladies and gentlemen, we’ve lost a third engine, but we’ll make it safely to Portland with the one engine we’ve got left. It’s just gonna take 12 hours.”
That was when the guy in the seat next to me lost it. “I sure hope we don’t lose that last engine,” he yelled, “or we’re gonna be up here all day!”
That story isn’t true. None of it. Not. One. Single. Fact. I mean, come on! Yellowknife? Why would I need to go to Yellowknife? To brush up on my Dogrib?
But the following story is true.
My honorary niece Ivy occasionally calls for help with math. Not surprisingly, the frequency of calls has increased with online learning during the pandemic. This is a modified version of a question about which she recently called:
A plane traveling against the wind traveled 2,460 miles in 6 hours. Traveling with the wind on the return trip, it only took 5 hours. What was the speed of the plane? What was the speed of the wind?
It’s assumed that the plane flew at the same average speed in both directions and that the wind was equally strong throughout. On a standardized test, those assumptions would need to be stated explicitly. But on this blog, I make the rules, and I refuse to add more words to explain reasonable assumptions without which the problem would be impossible to solve.
As presented to Ivy in an online homework assignment, the problem stated that x should represent the plane’s speed and y should represent the speed of the wind. My first question was, “Why? What’s wrong with p and w as the variables for plane and wind, respectively?” But my second, and perhaps more important, question was, “Why are they forcing algebra on a problem that is much easier without it?”
Somewhere, many years ago, someone decided that the methods of substitution and elimination were critically important, if not for success in life then at least for success in high school. Anecdotally, this seems to be true; I was a bad-ass systems-of-equations solver in high school, and I was also the captain of the track team that won the county title, so… yeah. Sadly, that someone seemed to put less stock in a slightly more critical skill: thinking.
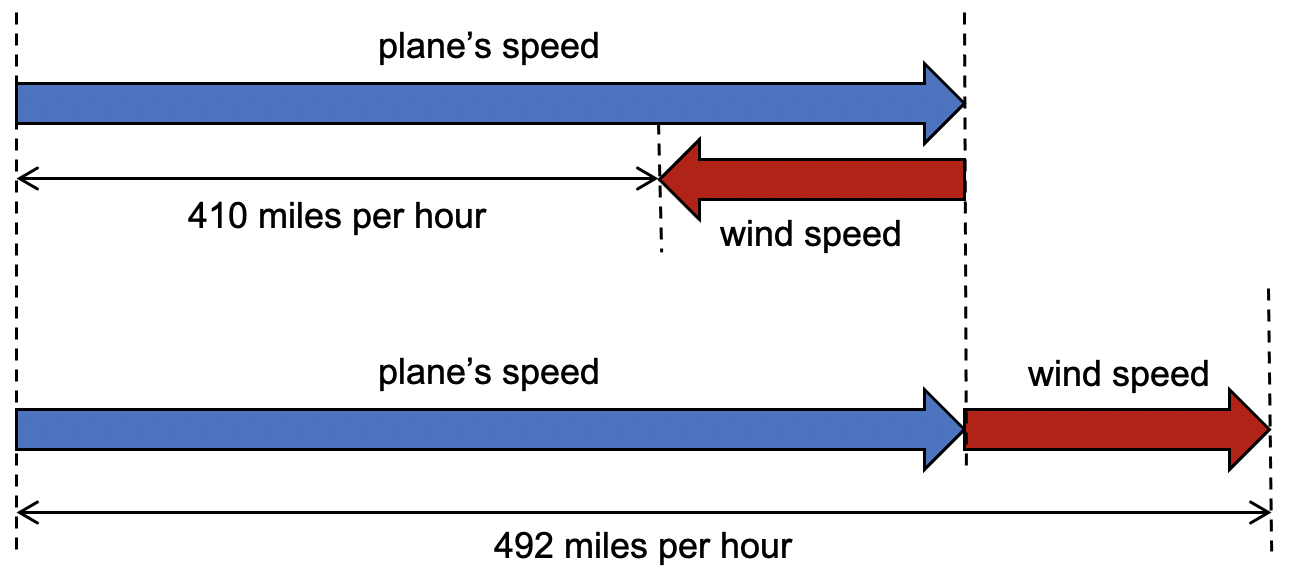
Here’s the deal. Against the wind, the plane covered 2,460 miles in 6 hours, so the plane’s speed on the way out was 410 miles per hour. With the wind, the plane covered those same 2,460 miles in just 5 hours, so the plane’s speed on the way back was 492 miles per hour. Since the wind is constant in both directions, the unaided speed of the plane must have been 451 miles per hour, exactly halfway between the against-the-wind and with-the-wind speeds. Visually,
The top portion of that diagram represents the plane flying against the wind; the bottom portion represents the plane flying with the wind. This makes it obvious that the the unaided speed of the plane is equidistant from 410 miles per hour and 492 miles per hour, and that distance is the speed of the wind.
Algebraically, the problem might be solved like this…
which is no different than the logical approach above: x ‑ y is the speed of the plane against the wind, and x + y is the speed of the plane with the wind, so the numbers are obviously the same. But using algebra just seems so much more… cumbersome.
Students in elementary classrooms often explain their thinking with drawings and diagrams. Unfortunately, some of that great thought is abandoned when students take a course called Algebra, and the mechanical skill of symbolic manipulation replaces the mathematical skill of logical reasoning. That’s a tragedy. As Jim Rubillo, former executive director at NCTM, used to say, “We have to stop being so symbol‑minded.”
Covering 100 Squares
There’s an old math joke that says math books are sad because they have too many problems. But I disagree; I believe that most math books — and, in particular, textbooks — are sad because they have too many exercises.
To try to release more true problems into the wild, I recently wrote a book called One-Hundred Problems Involving the Number 100. Published by NCTM, the book contains problems, suggestions for classroom use, and solutions. Some of the problems are old chestnuts, such as, “What is 1 + 2 + 3 + ⋯ + 100?” (Problem 21: Gauss and Check). Others are complete originals, and occasionally a little silly, such as, “If all the positive integers from 1 to 100 were spelled out, how many letters would be used?” (Problem 1: Spell It Out). But my favorite problem in the book, which I’ve shared twice during NCTM’s 100 Days of Professional Development (May 14, Oct 7; login required), is Problem 100: Covering with Squares.
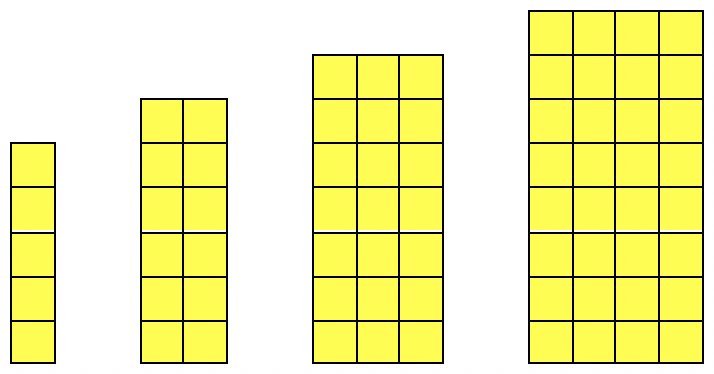
As shown below, a square grid with 100 smaller squares can be covered by 100 squares (each measuring 1 × 1), by 25 squares (each measuring 2 × 2), or by 13 squares (one 6 × 6, two 4 × 4, two 3 × 3, two 2 × 2, and six 1 × 1).
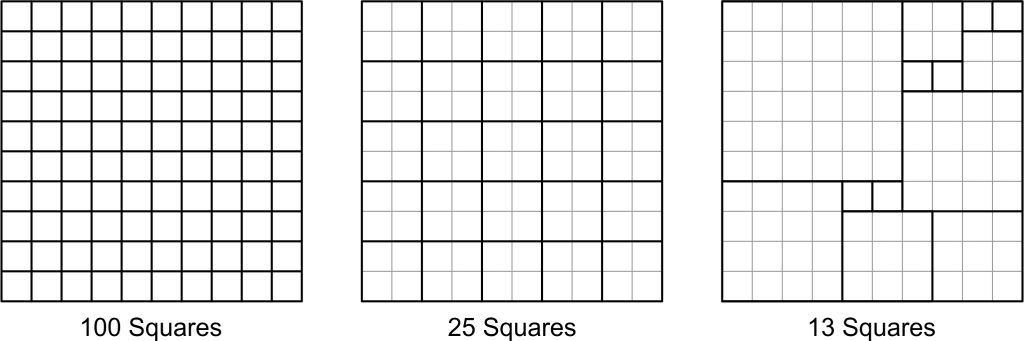
Find all values of 0 < n < 100 for which it is impossible to cover a 10 × 10 grid with n squares of integer side length.
I’ll admit, I’m not ecstatic about the phrasing of the question at the end. The symbolic representation makes it succinct, sure, but this problem can be investigated by students who may be too young to understand that notation. It might be better to just ask, “Can you cover it with two squares? Can you cover it with 58 squares? For how many different numbers of squares can you find a covering?”
You can, of course, explore this problem using graph paper. But for a digital experience, click on the link below and explore using Google Slides:
When you click on that link, you’ll be required to “Make a copy” for your own Google Drive. This is done for two reasons: first, it’ll prohibit any editing to the original file, so that other folks who use that link will receive a similarly pristine copy; and second, it places a copy in your Google Drive that you can play with, modify, or share with your students. If you do that, be sure to change /edit and anything that follows at the end of the URL to /copy, which will require anyone you share it with to make a copy, too. Of course, you could also just share the link above with your students, and they can have a copy of the original file.
When using this problem in the classroom, start by showing a few examples — such as the ones above — and then have all students find a covering for the grid that’s different from your examples. Because every student can find at least one covering, this breeds confidence. In addition, it ensures that all students understand the problem. When all students have found a covering, have them share it with a partner. From then on, allow students to work in pairs or small groups to find other coverings.
To facilitate the discussion, I draw a blank hundreds grid on the whiteboard. As unique coverings are found, students are allowed to enter the number in the hundreds grid. Of course, I require them to first verify with a partner that they have counted the number of squares correctly; once confirmed, they can show the covering to me; and if that number hasn’t been entered in the hundreds grid yet, they get to enter it. This can be motivating for students, and it’s a good opportunity to get less participatory students engaged.
When using this problem recently with a group of students, this is how the hundreds grid looked after about 20 minutes:
Whiteboard Record of Student Work
You’ll notice that numbers appear in different colors; each group received a different color dry erase marker. What you might also notice is that the numbers 17, 20, 23, 26, …, 65 all appear in red. This seemed more than coincidental, so I asked about it. The group members explained:
We realized we could cover the grid with a one 6 × 6 square and sixteen 2 × 2 squares:
We then divided one of the 2 × 2 squares into four 1 × 1 squares. That meant that one 2 × 2 square was replaced by four 1 × 1 squares, increasing the number of squares by three. So, the grid was now covered with 17 + 3 = 20 squares.
If we kept doing that — if we kept dividing the 2 × 2 squares — then we’d keep adding three more squares, so we could get 23, 26, 29, and so on, all the way up to 65.
The realization that one configuration could be transformed into many others allowed students to find coverings for myriad numbers. For what values of n could the grid not be covered? That’s left as a question for you. Have fun!
When a Half Is More Than a Half (and When It Ain’t)
Tonight, the dreadful Philadelphia Eagles defeated the pathetic New York Giants 22‑21 in a match-up of horrendous one-win teams. But not all one-win teams are created equal: in late September, the Eagles played the Bengals to a 23‑23 tie in a game that might have featured the all-time worst ending ever. As a result, the Eagles entered tonight’s game with a horrid 1‑4‑1 record, but not to be outdone, the Giants entered the game with a slightly more putrid 1‑5 record.
In football, a tie counts as a half-win (and a half-loss). But half-wins are sometimes worth more than half a win, sometimes they’re worth less than half a win, and sometimes they’re worth exactly half a win. Let me ‘splain.
After their win tonight, the Eagles record is 2‑4‑1. For the time being, that puts them atop the lowly NFC East:
| Eagles | 2-4-1 |
| Cowboys | 2-4 |
| Washington | 1-5 |
| Giants | 1-6 |
Philadelphia has played 7 games and won 2 1/2 of them. That is, they’ve won
of their games. That puts them ahead of Dallas, who has won
of their games. So, the Eagles are currently in first place by 1/42 of a game.
But let’s say the Eagles had entered tonight with a 3‑2‑1 record and the Cowboys were 4‑2. After tonight’s win, the Eagles would be 4-2-1, and they would’ve won
of their games. The Cowboys, on the other hand, would have won
of their games, and the Cowboys would be leading the division by 1/42 of a game.
So that half-win tie? It’s worth more to the Eagles because they’re terrible. Were they at least mediocre, that tie wouldn’t be as valuable.
On the other hand, if the Eagles had entered tonight with a 2‑3‑1 record and the Cowboys were 3‑3, then the Eagles would have been 3-3-1 after tonight’s win, and they would’ve won
of their games. Similarly, the Cowboys would have won
of their games, and the teams would’ve been tied for first in the pitiful, talentless, miserable NFC East.
(Yes, I’m being hard on the NFC East, but it isn’t unwarranted. The average power ranking of the four teams is 28, when the lowest possible is 30.5. The four starting quarterbacks have thrown nearly as many interceptions as touchdowns (24 TDs, 22 Ints), and the four teams’ top running backs have more fumbles than touchdowns (11 TDs, 12 Fum). Seriously, this division may be all-time bad.)
All that said, it’s highly unlikely that the season will end with the Eagles having played more games than the Cowboys. Then again, with COVID‑19, who knows what might happen?
It’s often been said that football is “a game of inches.” But given the importance of half-wins, isn’t it time we started saying that football is “a game of fractions”?
Words No Longer Used
I’ve been listening to the audiobook of At Home by Bill Bryson, and there’s a segment where he talks about words previously used to refer to the bathroom. My favorite is
necessarium
with its Latin meaning of “necessity,” implying that a room dedicated to urinating and defecating may not be something we really want in our house but very much need.
This made me wonder about mathematical words that are no longer in use. Many have gone the way of necessarium, but I think they deserve consideration for reintroduction. Well, maybe not all of them. Let’s have a look…
octothorpe, n. : another name for the pound sign (#); the hashtag. Wouldn’t it be great if #worldoctopusday were read as “octothorpe world octopus day”?
surd, n. : a square root that cannot be reduced further. This word comes from its meaning in phonetics of “mute” or “voiceless” for an unvoiced consonant; in math, it refers to an expression that cannot be expressed (spoken) as a rational number. The following radical would be ab‑surd:
vinculum, n. : a horizontal line drawn over a group of terms in a mathematical expression that serve as a grouping, such as the line on top of a radical that indicates the number for which the root is to be taken, or the fraction bar, which appears over the entire denominator. Still used occasionally, but rarely.
Logo of Vinculum, a global software company.
solidus, n. : the diagonal slash “/” used as the bar between numerator and denominator of an in-line fraction. Also, a famous Roman bodybuilder.
synonym diagonal
virgule, n. : a diagonal slash resembling the solidus, but with slightly less slant, used to denote division for in-line equations. This is also the name for the line used to indicate a choice between two terms in writing, e.g., and/or or pass/fail.
lattermath, n. : aftermath. Okay, not really a math term, but on the list since it contains “math.”
porism, n. : an archaic type of mathematical proposition whose historical purpose is not entirely known. It is used instead of “theorem” by some authors for a small number of results for historical reasons.
Jacob’s staff, n. : a mathematical instrument used for measuring heights and distances; typically, a pole with length markings on it.
anthyphairetic ratio, n. : a continued fraction, such as
Same number of syllables as parallelogram and inequality, but cooler than either of those. If you looked at anthyphairetic and thought, “that’s Greek to me,” you’d be entirely correct.
